This paper calculates how much control a craps shooter must possess on dice outcomes to eliminate the house advantage. A golden arm is someone who has dice control (or a rhythm roller or dice influencer). Aliante casino slot machines. There are various strategies for dice control in craps. We discuss several possibilities of dice control that would result in. Out of the countless possibilities, the most popular dice are included in the Dungeons & Dragons dice set, which contains seven different polyhedral dice: 4 sided dice, also known as a tetrahedron - each face is an equilateral triangle; 6 sided dice, a classic cube - each face is a square.
Craps Odds and Probabilities - Calculating Craps Combinations
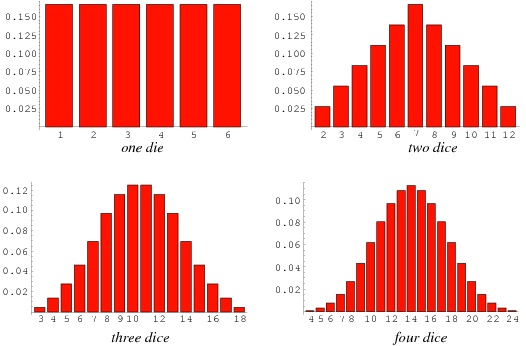
Calculating craps odds and probability seems hard, but it's not as complicated as one might think. When calculating the probabilities of any gambling activity, the first thing one looks at is the number of potential outcomes. Casino slot apps for android. When rolling two six-sided dice, like you in a game of craps, there are 36 possible outcomes. (There are only 11 possible totals, 2 through 12, but there are 36 combinations that can result in those totals.)
There is only one way to roll a 2 (or a 12). Roll a 1 on each die (or a 6 on each die.) Since there are 36 possible combinations, and only 1 of those combinations can total 2, the probability of getting a 2 on a roll is 1 out of 36, or 35 to 1, as stated in odds terms. There are 2 ways to roll a 3 though - you can roll a 1 and a 2, or roll a 2 and a 1, so the probability of rolling a 3 is 2 out of 36. 2 out of 36 is the same as 1 out of 18, which stated in odds terms is 17 to 1.
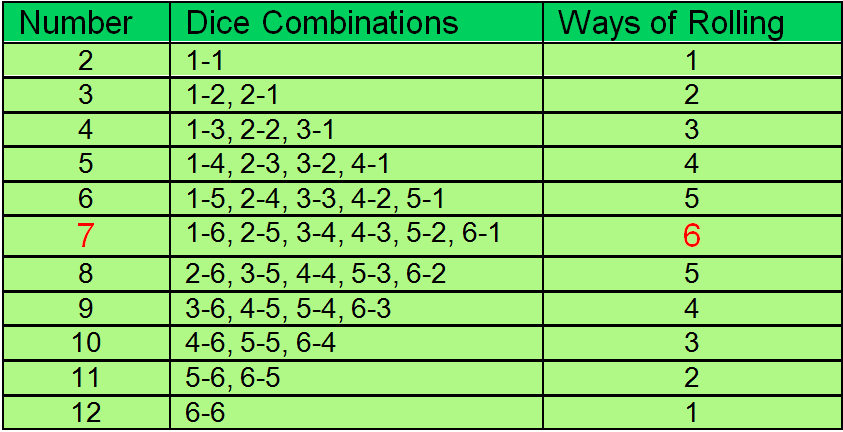
Here's a chart outlining the possible combinations, how many ways each total can be rolled, and what the odds are for each total.
| Total | Number of Ways to Roll This Total | Odds | Combinations |
| 2 | 1 | 35 to 1 | 1,1 |
| 3 | 2 | 17 to 1 | 1,2 + 2,1 |
| 4 | 3 | 11 to 1 | 1,3 + 3,1 + 2,2 |
| 5 | 4 | 8 to 1 | 1,4 + 4,1 + 2,3 + 3,2 |
| 6 | 5 | 6.2 to 1 | 1,5 + 5,1 + 2,4 + 4,2 + 3,3 |
| 7 | 6 | 5 to 1 | 1,6 + 6,1 +2,5 + 5,2 + 3,4 + 4,3 |
| 8 | 5 | 6.2 to 1 | 2,6 + 6,2 + 3,5 + 5,3 +4,4 |
| 9 | 4 | 8 to 1 | 3,6 + 6,3 + 4,5 +5,4 |
| 10 | 3 | 11 to 1 | 4,6 + 6,4 + 5,5 |
| 11 | 2 | 17 to 1 | 5,6 + 6,5 |
| 12 | 1 | 35 to 1 | 6,6 |
Calculating the Odds in Craps
The formula used to calculate the odds of rolling a specific total in craps is actually pretty simple. Divide 36 by the number of combinations that will make that total. Since there are 6 combinations which will total 7, the probability is 36 divide by 6, or 1 in 6 chance of rolling a 7.
Converting this to odds is easy to. Odds are always stated as the number of possibilities of something not happening versus the number of possibilities of something happening. For every roll of 7 there will be 5 rolls that aren't 7.
Craps Probabilities and the House Edge
The house edge is the difference between what the house pays out on a bet and it's true odds. For example, if a casino pays $30 for a $1 bet that someone will roll a 2, it's making a profit, because the true odds are 35 to 1. To be a break even bet, the casino would need to pay out $35 on that bet. (And return the $1 bet.) But if a casino had nothing but break even bets, it wouldn't make a profit. Without a profit, they'd have no reason to exist, and one wouldn't get to play craps.

Craps Dice Odds Chart

Here's a chart outlining the possible combinations, how many ways each total can be rolled, and what the odds are for each total.
| Total | Number of Ways to Roll This Total | Odds | Combinations |
| 2 | 1 | 35 to 1 | 1,1 |
| 3 | 2 | 17 to 1 | 1,2 + 2,1 |
| 4 | 3 | 11 to 1 | 1,3 + 3,1 + 2,2 |
| 5 | 4 | 8 to 1 | 1,4 + 4,1 + 2,3 + 3,2 |
| 6 | 5 | 6.2 to 1 | 1,5 + 5,1 + 2,4 + 4,2 + 3,3 |
| 7 | 6 | 5 to 1 | 1,6 + 6,1 +2,5 + 5,2 + 3,4 + 4,3 |
| 8 | 5 | 6.2 to 1 | 2,6 + 6,2 + 3,5 + 5,3 +4,4 |
| 9 | 4 | 8 to 1 | 3,6 + 6,3 + 4,5 +5,4 |
| 10 | 3 | 11 to 1 | 4,6 + 6,4 + 5,5 |
| 11 | 2 | 17 to 1 | 5,6 + 6,5 |
| 12 | 1 | 35 to 1 | 6,6 |
Calculating the Odds in Craps
The formula used to calculate the odds of rolling a specific total in craps is actually pretty simple. Divide 36 by the number of combinations that will make that total. Since there are 6 combinations which will total 7, the probability is 36 divide by 6, or 1 in 6 chance of rolling a 7.
Converting this to odds is easy to. Odds are always stated as the number of possibilities of something not happening versus the number of possibilities of something happening. For every roll of 7 there will be 5 rolls that aren't 7.
Craps Probabilities and the House Edge
The house edge is the difference between what the house pays out on a bet and it's true odds. For example, if a casino pays $30 for a $1 bet that someone will roll a 2, it's making a profit, because the true odds are 35 to 1. To be a break even bet, the casino would need to pay out $35 on that bet. (And return the $1 bet.) But if a casino had nothing but break even bets, it wouldn't make a profit. Without a profit, they'd have no reason to exist, and one wouldn't get to play craps.
Craps Dice Odds Chart
Craps Dice Odds
It's good to understand how probabilities and odds are calculated because not all bets have as high a house edge as other bets. If one understands the math behind the odds, one can then choose the better bets, the ones with the lowest house edge. Choosing the bets with the lowest house edge is just good craps strategy.